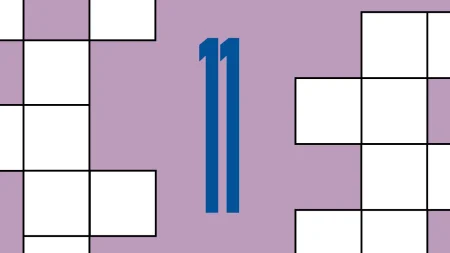
James Foster’s zero-less number system, introduced in 1947, challenges the perceived indispensability of zero in our numerical understanding. While zero signifies the absence of a particular place value, Foster’s system avoids this need by delaying the “boxing up” of values into higher place values. In our decimal system, when we reach ten units, we group them into a single ten and represent the absence of units with a zero. Foster’s system, however, continues accumulating units until the eleventh arrives, representing ten as “T.” This principle applies to all place values, effectively eliminating the need for a zero symbol. This system doesn’t reinvent numbers but offers a parallel approach to representing quantities, demonstrating that zero, while incredibly useful, isn’t fundamentally necessary for a functional number system.
Foster’s system operates on a delayed grouping principle. Imagine collecting items. In our standard base-10 system, we group ten items into a single unit of the next higher place value. Foster’s system postpones this grouping. We continue collecting individual units until we have ten. We represent this ten with ‘T.’ We then continue collecting units until we reach another ten, resulting in ‘TT’ indicating twenty. Only when we acquire an additional unit, reaching twenty-one, do we group the two tens into the next higher place value. This process repeats for every place value, effectively sidestepping the need for a zero to indicate an empty place value.
The implications of this system ripple through the representation of all numbers containing zeros. Familiar numbers like 30 become 2T (two tens and another ten), while 106 transforms into T6 (ten tens plus six units). Larger numbers like 3,090 become 2T8T (two thousands, eight tens, and another ten). While appearing unconventional, this system maintains logical validity, mirroring our base-10 system in its core functionality. The absence of zero requires a shift in how we pronounce and interpret these numerical representations, leading to terms like “ten-teen” for 1T (20 in our system) and “ten-ty six” for T6 (106 in our system). This highlights how deeply ingrained the concept of zero is in our numerical thinking.
The absence of zero in Foster’s system compels a reevaluation of how we perceive and interact with numbers. While numbers without zeros remain unchanged, the presence of zeros in our conventional system requires a complete reimagining of their representation in Foster’s system. This prompts us to reconsider the significance we attach to certain numerical milestones. For instance, a “six-figure salary,” conventionally signifying a high income, may no longer hold the same weight if, say, $100,000 is represented as TTTTT. Anniversaries and milestones like 100 years or the 1,000th customer would be marked differently, requiring adjustments in our cultural perception of numerical significance.
Furthermore, the practical implications of a zero-less system extend to various aspects of daily life. Odometer readings, scoring systems in sports, and even the way we express dates and times would require adjustment. Imagine a basketball game where Wilt Chamberlain scored 9T points instead of the historic 100. While the achievement remains the same, the representation shifts our perception. The year 2024 would become 1T14, prompting us to reevaluate how we perceive historical timelines and the passage of time. This thought experiment underscores the pervasive influence of zero in shaping our understanding of numerical quantities and their significance.
Ultimately, Foster’s zero-less system serves not as a practical replacement for our established numerical system but as a powerful intellectual exercise. It challenges the assumed essentiality of zero, demonstrating that alternative systems can achieve the same functionality through different means. While Foster’s system may appear cumbersome and less intuitive, its very existence forces us to appreciate the elegance and efficiency that zero brings to our mathematical operations and our understanding of the world around us. It highlights the profound impact of zero, a symbol representing nothingness, on our ability to represent and manipulate quantities, emphasizing its crucial role in the development of mathematics and the sciences. The exploration of alternative systems like Foster’s allows us to gain a deeper appreciation for the ingenuity and impact of our familiar numerical conventions.